Similarly, if there is enough information to lead to a definite no, then you can also take decisive action. The only time trouble arises is when the information could lead to a yes or to a no; this situation leaves you in a position where you may have to guess.
Since the GMAT gives you two statements to work with, the appeal of using both statements in any given situation is pretty high. Statement 1 gives you some information, and statement 2 gives you more information. Everything provided must be accurate, although it may or may not be helpful. This leads to a very common bias: Wanting to pick both statements as the correct answer choice. Because the answer choice that uses both statements together is invariably answer choice C, this construct is known as the C-trap. (It’s a trap!)
The reason this trap is so tempting is that both statements together frequently give you exactly the correct answer with little or no variability in the data. In other words, both answer choices often narrow down the answer to one specific value, which may or may not be more specific than what the answer is looking for. This is understandably enticing, but answer choice C specifically says “Both statements 1 and 2 together are sufficient to answer the question but neither statement is sufficient alone.” So the two statements together may give the correct answer, but either statement alone must also be insufficient in order for C to be the correct choice.
The way I like to explain this is that each statement costs you $1 M, and getting the right answer to the question gets you $10 M. If you get the right answer using both statements, then you’ll spend $2 M to make $10 M, which means you made a profit of $ 8 M. You’re happy. However if you could have made a profit of $9 M by only paying for one statement, you just left a million dollars on the table. If you do that, your boss won’t be happy with you. Even if you feel like you have the right answer to the question, you have squandered a sizable chunk of change.
As an example, let’s look at a perfect example of a C-trap question:
Of the 60 families in a certain neighborhood, 38 have a cat. How many families in this neighborhood have a dog?
(1) 28 of the families in the neighborhood have a cat but not a dog
(2) The number of families in the neighborhood who have a dog and a cat is the same as the number of families who have neither a cat nor a dog.
(A) Statement 1 alone is sufficient but statement 2 alone is not sufficient to answer the question asked.
(B) Statement 2 alone is sufficient but statement 1 alone is not sufficient to answer the question asked.
(C) Both statements 1 and 2 together are sufficient to answer the question but neither statement is sufficient alone.
(D) Each statement alone is sufficient to answer the question.
(E) Statements 1 and 2 are not sufficient to answer the question asked and additional data is needed to answer the statements.
If you don’t look at the statements one at a time, you could easily look at this and determine that both statements together give you everything you could want. Statement 1 tells you 28 many families have only cats, which combined with the 38 families with cats given in the question stem yields the number of households that have both cats and dogs (10). Statement 2 then tells you that the number of families with both cats and dogs is the same as the number of families with neither. This can be visually represented via Venn diagram.
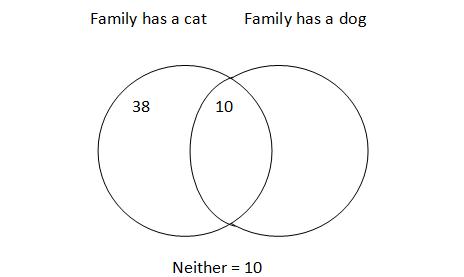
Together, both statements tell you how many families have cats, both cats & dogs and neither cats nor dogs. Since you know the total number of families, that means you have every single piece of information required in the Venn diagram formula except for one, which means you can solve it algebraically.
Since Total = Group 1 + Group 2 – Both + Neither, we know
60 = 38 + Group 2 – 10 + 10.
Solving for Group 2, it must equal exactly 22 families. This means both statements together definitely give us all the information we need to get the correct answer. However this only really eliminates answer choice E, as we have not evaluated the answer choices alone. The correct answer could still be A or B, as combining them could be overpaying for information.
Statement 1 on its own tells you that there are 10 families with both a cat and a dog, but it doesn’t give you any indication about how many families have neither a cat nor a dog. You could easily have 10 families with both and 0 with neither, which would leave (60 = 38 + x – 10 + 0) 32 families with a dog. Playing devil’s advocate, if one family had neither animal, then there would be (60 = 38 + x – 10 + 1) 31 families with a dog. We already have two different answers, and can therefore eliminate statement 1 alone, which means the answer is neither A nor D.
Evaluating statement 2 alone, the number of families with both is equal to the number of families with neither. At first glance, this doesn’t seem to give us any numbers, so it’s hard to imagine this statement being sufficient. However, thinking about this algebraically, the statement guarantees that Both = Neither, regardless of what the actual value is. If we consider this value as Y and look at our general Venn diagram formula:
Total = Group 1 + Group 2 – Both + Neither
Or: 60 = 38 + X – Y + Y.
Regardless of the value of Y, it will cancel out and leave us with:
60 = 38 + X.
Therefore, no matter how many families have no pets or both kinds, since they always cancel each other out, the number of families with dogs must be 22. Answer choice B gives us enough information to solve this question independently of statement 1, and therefore we can solve this question using only statement 2. Just like the example given above, you never want to overpay for the same information. If you can get the right answer using only one statement, why spend the extra million on a superfluous statement. Avoiding the tempting C-trap will lead to more correct answers on Data Sufficiency, and that’s advice you can take to the bank.
This article is part of the reading material along with online instruction, videos, and a live Q&A of a Free Online Live GMAT Course. Find details and join here.




